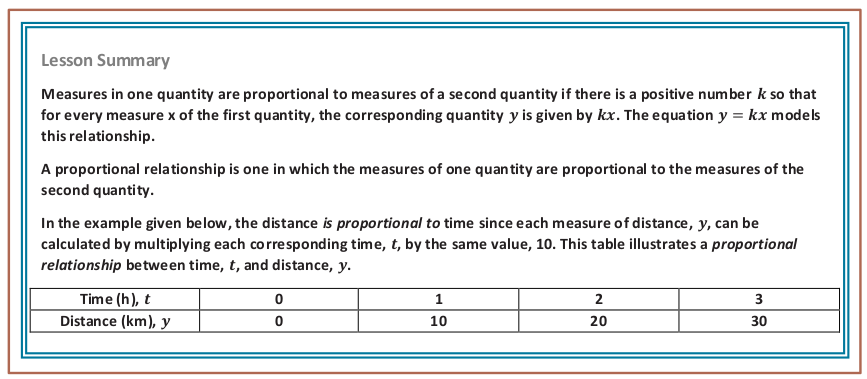
[expand title=”Warm Up”]
[/expand]
[expand title=”Lesson”]
Example 1 Continued – Discussion
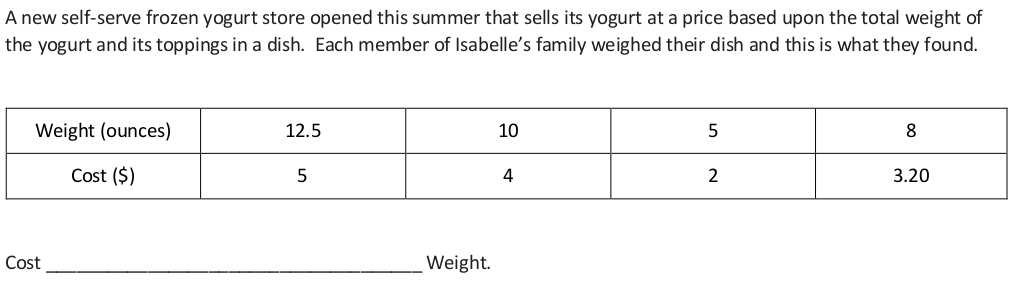
- Does everyone pay the same cost per ounce? How do you know?
- Isabelle’s brother takes an extra-long time to create his dish. When he puts it on the scale, it weighs 15 ounces.
If everyone pays the same rate in this store, how much will his dish cost? How did you calculate this cost?
- What happens if you don’t serve yourself any yogurt or toppings, how much do you pay?
- And does the relationship above still hold true? In other words, if you buy 0 ounces of yogurt, then multiply by
the cost per ounce, do you get 0?
- You always multiply the number of ounces, x, by the constant that represents cost per ounce, to get the total
cost, y.
S.4 Example 2
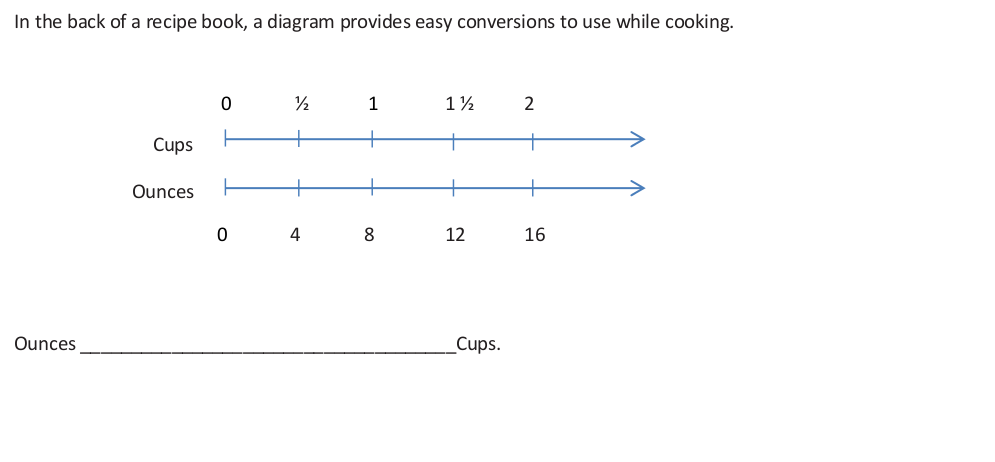
- What does the diagram tell us?
- Is the number of ounces proportional to the number of cups? How do you know?
- How many ounces are there in 4 cups? 5 cups? 8 cups? How do you know?
- Adding x and y variables – For any number of cups x, how do we find the number of ounces, y?
- Can we write an expression in terms of x and y?
- How can we verify our equation?
S.4 Exercise 1 (Independent Work)
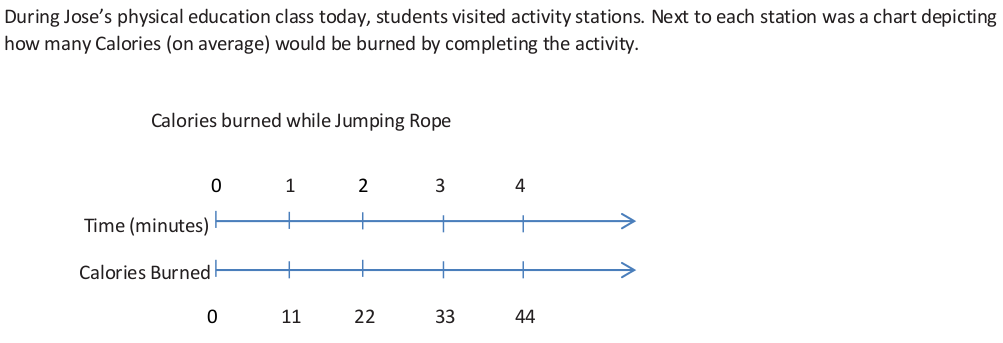
1. Is the number of Calories burned proportional to time? How do you know?
2. If Jose jumped rope for 6.5 minutes, how many calories would he expect to burn?
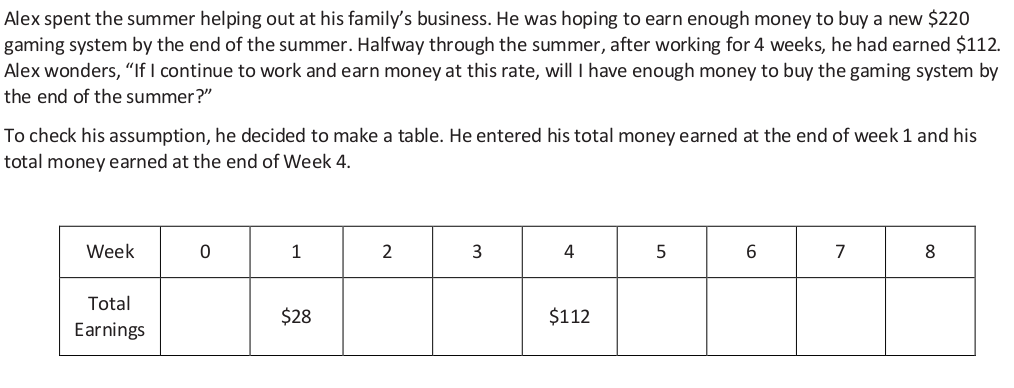
1. Work with a partner to answer Alex’s question.
2. Are Alex’s total earnings proportional to the number of weeks he worked? How do you know?
[/expand]
[expand title=”Summary”]
- How do we know if two quantities are proportional to each other?
Two quantities are proportional to each other if there is one constant number that is multiplied by each
measure in the first quantity to give the corresponding measure in the second quantity.
- How can we recognize a proportional relationship when looking at a table or a set of ratios?
If each of the measures in the second quantity is divided by its corresponding measure in the first
quantity and it produces the same number, called a constant, then the two quantities are proportional to each other.
[/expand]
[expand title=”Problems”]
S.7
[/expand]
[expand title=”Exit Ticket”]
Ms. Albero decided to make juice to serve along with the pizza at the Student Government party. The directions said to
mix 2 scoops of powdered drink mix with a half a gallon of water to make each pitcher of juice. One of Ms. Albero’s
students said she will mix 8 scoops with 2 gallons of water to get 4 pitchers. Is the student is correct?
[/expand]