Essential Question: How do we examine situations to determine whether two quantities are proportional?
7.RP.2b
7.RP.2d
[expand title=”Warm up”]
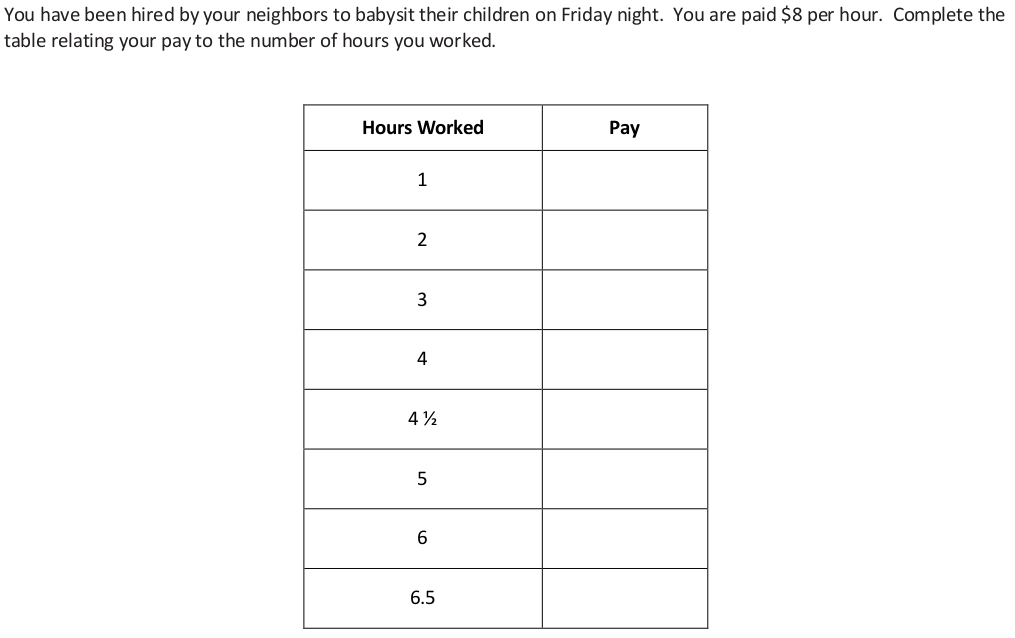
S.8
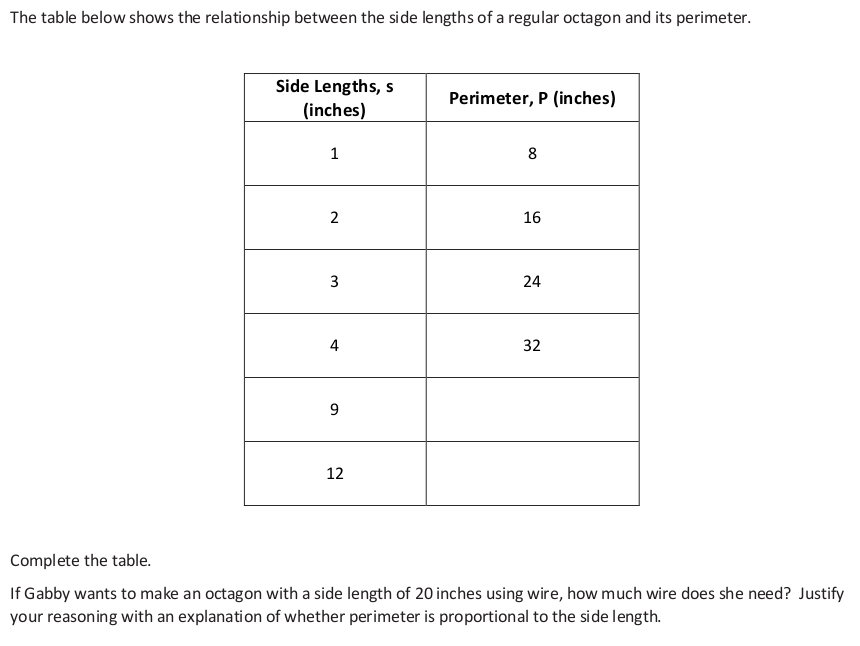
1. Based on the table above, is pay proportional to hours worked? How do you know?
[/expand]
[expand title=”Lesson 3″]
Discussion
- Explain how you completed the table
- How did you determine the pay for 4.5 hours?
- How could you use the information to determine the pay for a week in which you worked 20 hours?
- If the quantities in the table were graphed, would the point (0,0) be on that graph? What would it mean in the context of the problem?
- For each example, if the quantities in the table were graphed, would the point (0,0) be on that graph? Describe what the point (0,0) would represent in each table.
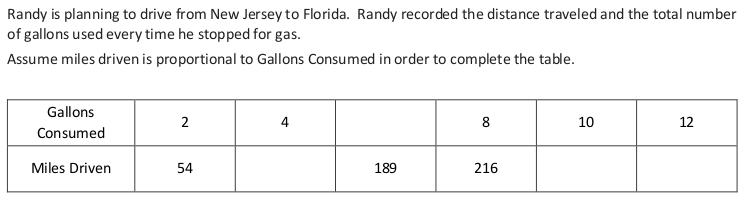
- Why is it important for you to know that miles are proportional to the gallons used?
- Describe the approach you used to complete the table.
- What is the value of the constant? Explain how the constant was determined.
[/expand]
[expand title=”Summary”]
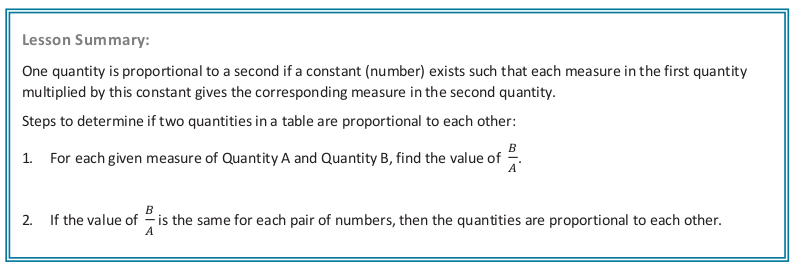
How can you use a table to determine whether the relationship between two quantities is proportional? The
quantities are proportional if a constant number exists such that each measure in the first quantity multiplied by
this constant gives the corresponding measure in the second quantity.
[/expand]
[expand title=”Problems”]
S.11
[/expand]
[expand title=”Exit”]
[/expand]
[expand title=”Warm up”]
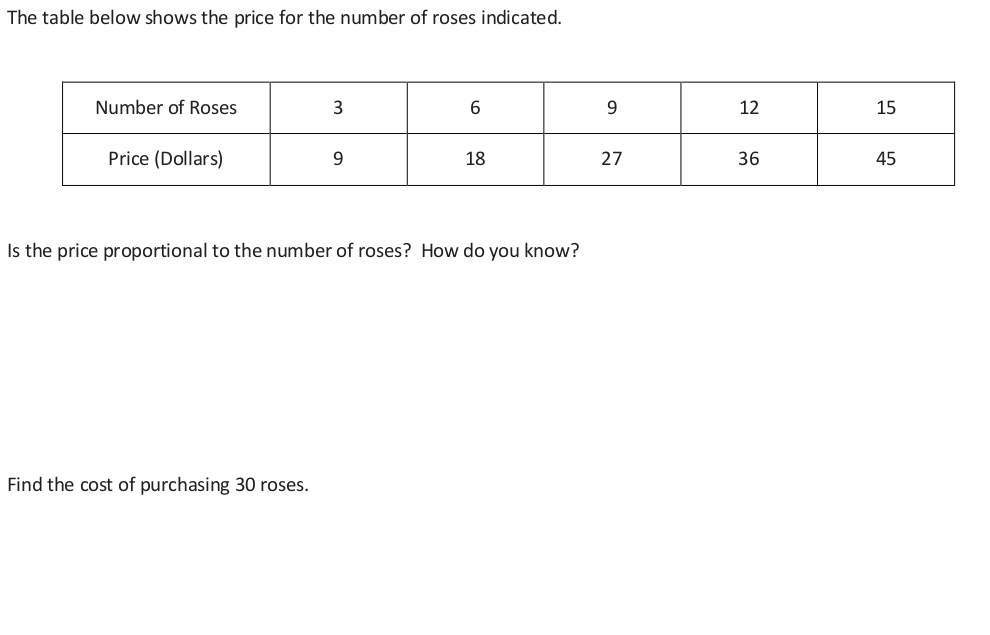
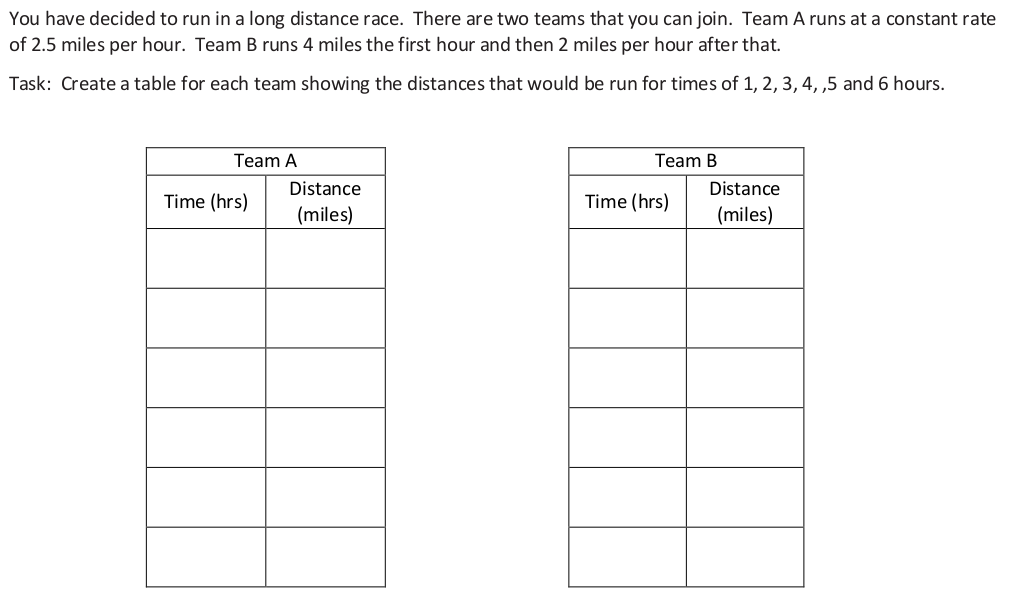
![]() S.13 Example 1 Complete both tables (Independent Work)
S.13 Example 1 Complete both tables (Independent Work)
[/expand]
[expand title=”Lesson 4″]
Discussion
1. For which team is distance proportional to time? Explain your reasoning.
2. Explain how you know the distance for the other team is not proportional to time.
3. If the race were 2.5 miles long, which team would win? Explain.
4. If the race were 3.5 miles long, which team would win? Explain.
5. If the race were 4.5 miles long, which team would win? Explain.
6. For what length race would it be better to be on Team B than Team A? Explain.
7. Using this relationship, if the members on the team ran for 10 hours, how far would each member run on each team?
8. Will there always be a winning team, no matter what the length of the course? Why or Why not?
9. If the race were 12 miles long, which team should you choose to be on if you wish to win? Why would you choose this team?
10. How much sooner would you finish on that team compares to the other team?
[/expand]
[expand title=”Problems”]
S.15
[/expand]
[expand title=”Exit”]
[/expand]