[expand title=”How can I simplify it?”]
Mathematics can be used to describe patterns in the world. Scientists use math to describe various aspects of life, including how cells multiply, how objects move through space, and how chemicals react. Often, when scientists try to describe these patterns, they need to describe something that changes or varies. Scientists call the quantities that change variables, and they represent them using letters and symbols.
In this course, you will spend time learning about variables, what they can represent, and how they serve different purposes. To start, you will use variables to describe the dimensions and areas of different shapes. You will begin to organize the descriptions into algebraic expressions.
As you work with your teammates, use these questions to help focus your team’s discussion:
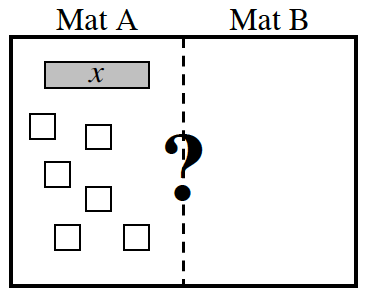
4-70. AREA OF ALGEBRA TILES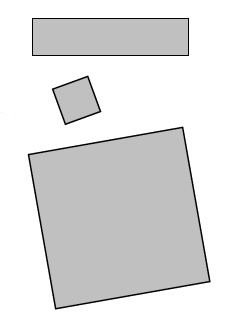
Your teacher will provide your team with a set of algebra tiles. Remove one of each shape from the bag and put it on your desk. Trace around each shape on your paper. Look at the different sides of the shapes.
a. With your team, discuss which shapes have the same side lengths and which ones have different side lengths. Be prepared to share your ideas with the class. On your traced drawings, color-code lengths that are the same.
b.Each type of tile is named for its area. In this course, the smallest square will have a side length of 1 unit, so its area is 1 square unit. This tile will be called “one” or the “unit tile.” Can you use the unit tile to find the side lengths of the other rectangles? Why or why not?
c.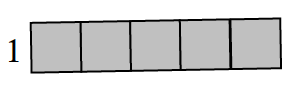 
If the side lengths of a tile can be measured exactly, then the area of the tile can be calculated by multiplying these two lengths together. The area is measured in square units. For example, the tile below measures 1 unit by 5 units, so it has an area of 5 square units.
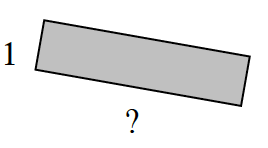The next tile below has one side length that is exactly one unit long. If the other side length cannot have a numerical value, what can it be called?
d. If the unknown length is called “x,” label the side lengths of each of the four algebra tiles you traced. Find each area and use it to name each tile. Be sure to include the name of the type of units it represents.
4-71. When a collection of algebra tiles is described with mathematical symbols, it is called an algebraic expression. Take out the tiles shown in the picture below or use 4-71 tiles (CPM). Then work with your team to do the following tasks
Use mathematical symbols (numbers, variables, and operations) to record the area of this collection of tiles.
Write at least three different algebraic expressions that represent the area of this tile collection.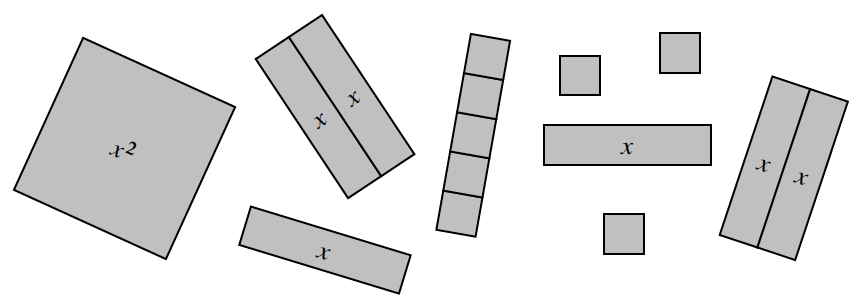 
4-72. Put the tiles pictured in each collection below on your table. Then work with your team to find the area as you did in problem 4-71.
a.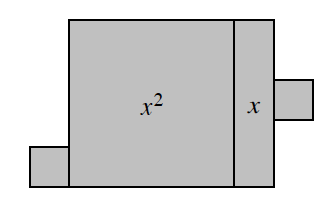 
b. 
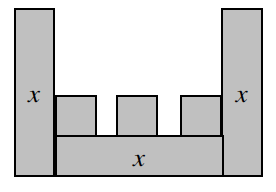
4-73. The perimeter of each algebra tile can be also written as an expression using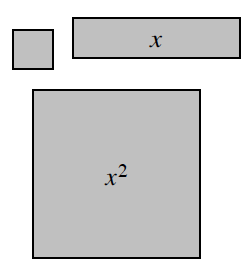 
variables and numbers.
a. Write at least two different expressions for the perimeter of each tile shown at right.
b. Which way of writing the perimeter seems clearest to you? What information can you get from each expression?
c. Lianna wrote the perimeter of the collection of tiles below as 2x + 1 + 1 + 1 + 2x + 1 units, but her teammate Jonah wrote it as 4x + 4. How are their expressions different?
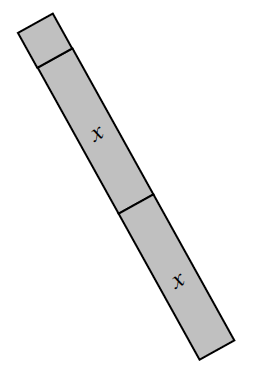
d. Which expression represents the perimeter?
4-74. The expressions that you have written to represent area and perimeter are made up of terms that are separated by addition and subtraction.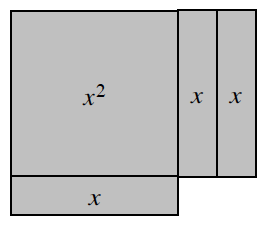
Write an expression for the perimeter of the figure at right.
a. How many x lengths are represented in the expression in part (a)? How many unit lengths?
b. Combining like terms (like terms contain the same variable raised to the same power) is a way of simplifying an expression. Rewriting the perimeter of the shape above as P = 4x + 6 combines the separate x‑terms as 4x and combines the units in the term 6.
If you have not already done so, combine like terms for the perimeter expressions that you wrote in problem 4-73.
Assignment: 4.3.1 Homework
[/expand]
[expand title=”How can I group them?”]
In Lesson 4.3.1, you used variables to name lengths that could not be precisely measured. Using variables allows you to work with lengths that you do not know exactly. Today you will work with your team to write expressions for the perimeters of different shapes using variables. As you work with your teammates, use these questions to help focus your team’s discussion:
4-85. Using algebra tiles on your desk, make the shapes shown below. Trace each shape and label the length of each side on your drawing. With your team, find and record the total perimeter and area for each shape. If possible, write the perimeter in more than one way.
a.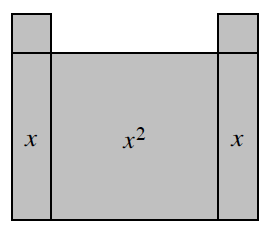 
b. 
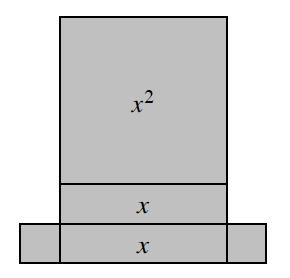c. 
4-86. In problem 4-85, x is a variable that represents a number of units of length. The value of x determines the size of the perimeter and area of the shape.
Using the shapes from problem 4-85, sketch and label each shape with the new lengths given below. Then evaluate each expression for the given value of the variable. That is, rewrite the expressions, replacing the variable with the number given, and then simplify them to determine the perimeter and area of each shape.
a. x = 6 for all three shapes
b. x = 
for all three shapes
c Compare your method for finding perimeter and area with the method your teammates used. Is your method the same as your teammates’ methods? If so, is there a different way to find the perimeter and area? Explain the different methods.
4-87. Build each of the shapes below using algebra tiles or explore using 4-87i tiles, 4-87ii tiles (CPM). Look carefully at the lengths of the right sides.
i. 
ii.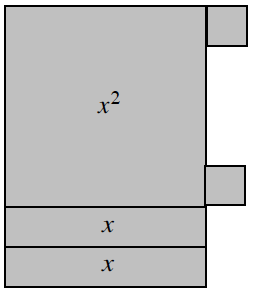 
a. Discuss with your team how to label the length of the right side of each figure. Label each length on your paper. Explain your reasoning.
b. Find the perimeter of each figure. Write the perimeter in simplest form by combining the like terms.
4-88. Build the shape at right using algebra tiles. Then, on graph paper, draw the shape when x is equal to each of the lengths below. Explore drawing the figures below using the algebra tiles.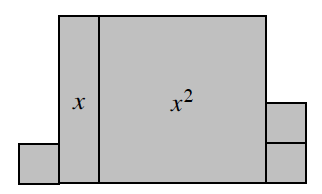
- x = 5 units
- x = 3 units
- x = 2 units
- x = 1 unit
4-89. Parentheses in an algebraic expression allow you to show that tiles are grouped together.
a. Build these steps with algebra tiles. Use an x tile to represent “any number.”
1. Think of any number.
2. Triple it.
3. Add 1.
4. Multiply by 2.
b. Look at the algebra tiles you used to build the final step of part (a). Write two different algebraic expressions to represent those tiles.
4-90. Build the following expressions with algebra tiles. Then rewrite the expression a different way. Remember that parentheses in an algebraic expression allow you to show that tiles are grouped together.
a. 4(2x + 3)
b. 12x + 18
c. 10 + 15x (Hint: Divide into as many equally-sized groups as possible)
4-91. You have been writing expressions in different ways to mean the same thing. These expressions depend on whether you see tiles grouped (like four sets of 2x + 3 in part (a) of problem 4-90) or whether you see separate tiles (eight x-tiles and 12 unit tiles). These two expressions are equivalent based on a mathematical property called the Distributive Property.
Use the Distributive Property to write an equivalent expression for 21x + 7. See if you can do it by visualizing tiles.
4-92. LEARNING LOG
In your Learning Log, explain what you know now about the Distributive Property and how to use it to write equivalent expressions. Include an example of different ways to write an expression with numbers and with variables, such as 3(x + 4) by drawing pictures and writing equivalent expressions. Title this entry “Distributive Property” and include today’s date.
Assignment:
[/expand]
[expand title=”How do these compare?”]
In Chapter 4, you worked with writing and simplifying expressions. As you wrote expressions, you learned that it was helpful to simplify them by combining like terms and removing zeros. In this lesson, you and your teammates will use a tool for comparing expressions. The tool will allow you to determine whether one expression is greater than the other or if they are equivalent ways of writing the same thing (that is, if they are equal).
Remember that to represent expressions with algebra tiles, you will need to be very careful about how positives and negatives are distinguished. To help you understand the diagrams in the text, the legend at right will be placed on every page containing a mat. It shows the shading for +1 and −1. This model also represents a zero pair.
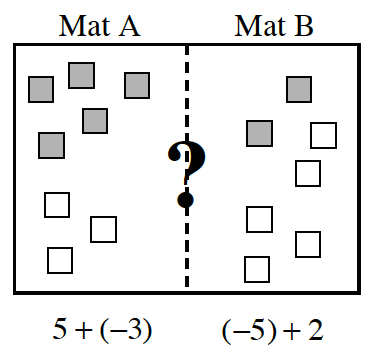
6-1. COMPARING EXPRESSIONS
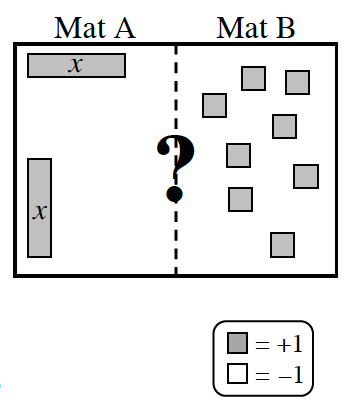
Ignacio and Oliver were playing a game. Each of them grabbed a handful of algebra tiles. They wanted to see whose expression had the greater value.
Two expressions can be compared by dividing the expression mat in half to change it into an Expression Comparison Mat. Then the two expressions can be built side by side and compared to see which one is greater.
- Oliver put his tiles on Mat A in the picture above and described it as 5 + (−3).
- Ignacio put his tiles on Mat B and said it was (−5) + 2.
With your team, find two different methods to simplify the two expressions so you can compare them. Which side of the mat is larger? Use your expression mat.
6-2. Using your Expression Comparison Mat, build the two expressions below using algebra tiles. Find a way to determine which side is greater, if possible. Show your work by sketching it on the Lesson 6.1.1B Resource Page. Be ready to share your conclusion and your justification. Explore using the 6-2 tiles (CPM).
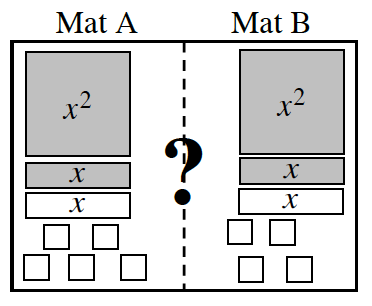
6-3. MORE COMPARING EXPRESSIONS – Is one expression greater?
Consider how you were able to compare the expressions in the previous problems. When is it possible to remove tiles to compare the expressions on the mats? In this problem, you will work with your team to identify two different “legal moves” for simplifying expressions.
Build the mat below using algebra tiles and simplify the expressions. Record your work by drawing circles around the zeros or the balanced sets of tiles that you remove in each step on the Lesson 6.1.1B Resource Page. Which expression is greater?
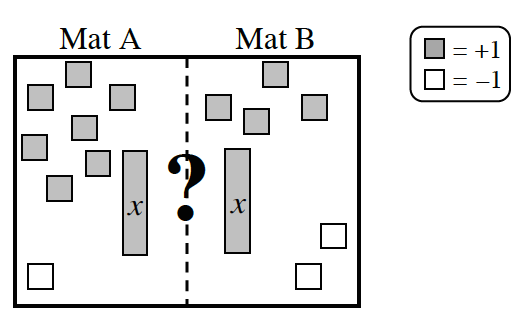
6-4. There are two kinds of moves you could use in problem 6-3 to simplify expressions with algebra tiles. First, you could remove matching (or balanced) sets of tiles from both sides of the mat. Second, you could remove zeros. Both moves are shown in the figures below. Justify why each of these moves can be used to simplify expressions.
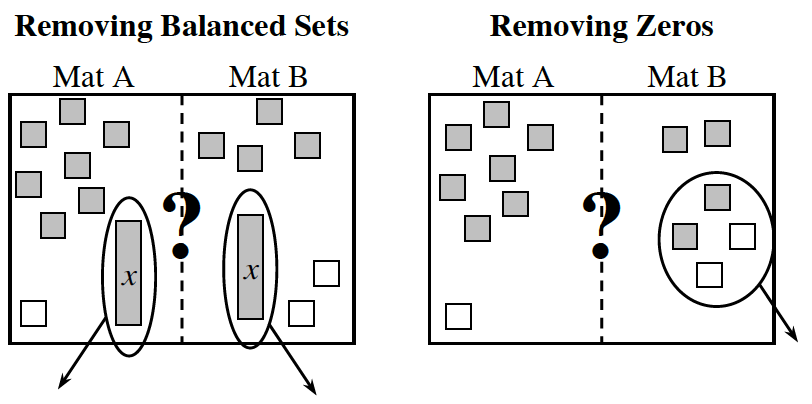
6-5. WHICH SIDE IS GREATER?
For each of the problems below, use the Expression Mat and:
- Build the two expressions on your mat using algebra tiles.
- Write an expression for each side below the mats for parts (a) through (d) OR draw the tiles in the space given on the resource page for parts (e) and (f).
- Use legal moves to determine which mat is greater, if possible. Record your work by drawing circles around the zeros or the balanced (matching) sets of tiles that you remove in each problem.
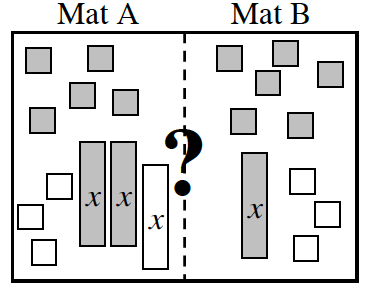
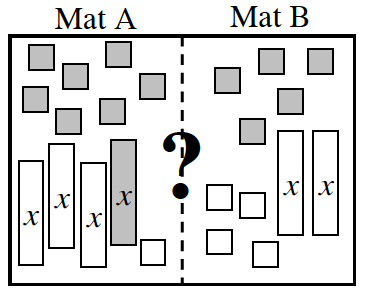
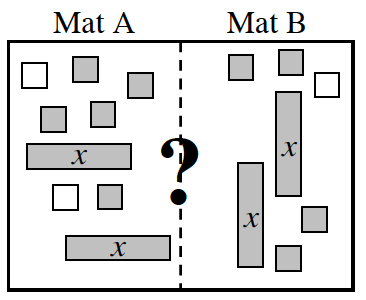
- Mat A: 3x − 4 − 2
Mat B: 3(x − 1) - Mat A: 5 + (−3x) + 5x
Mat B: x2 + 2x + 1 − x2
Assignment: 6.1.1 Homework
[/expand]
[expand title=”What if I cannot tell?”]
Have you ever tried to make a decision when the information you have is uncertain? Perhaps you have tried to make plans on a summer day only to learn that it might rain. In that case, your decision might have been based on the weather, such as, “I will go swimming if it does not rain, or stay home and play video games if it does rain.” Sometimes in mathematics, solutions might depend on something you do not know, like the value of the variable. Today you will study this kind of situation.
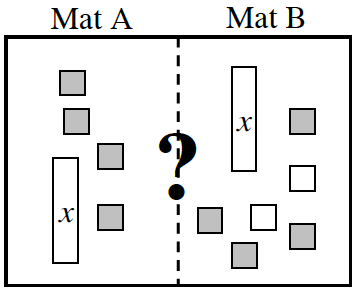
6-12. For each of the problems below, build the given expressions on your Expression Comparison Mat using algebra tiles. Then use the simplification strategies of removing zeros and simplifying by removing matching pairs of tiles to determine which side is greater, if possible. Record your steps on the Lesson 6.1.2 Resource Page.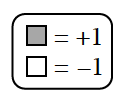
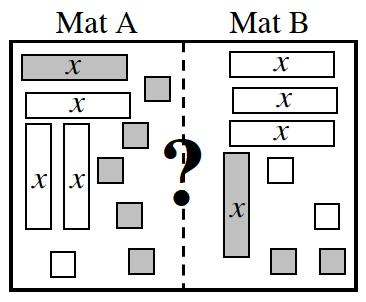
- Mat A: 2(x + 3) − 4
Mat B: 3x + (−1) − x + 4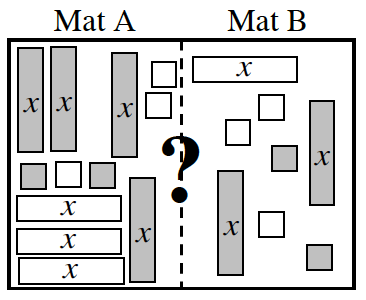 
6-13. WHAT HAPPENED?
When Ignacio and Oliver compared the expressions in part (d) of problem 6-12, they could not figure out which side was greater.
- Is it always possible to determine which side of the Expression Comparison Mat is greater (has the greater value)? Why or why not? Be prepared to share your reasoning.
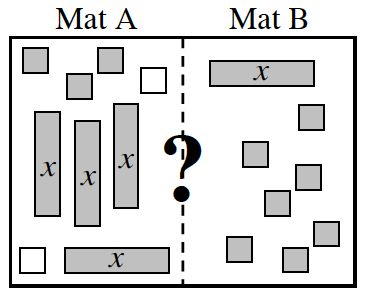
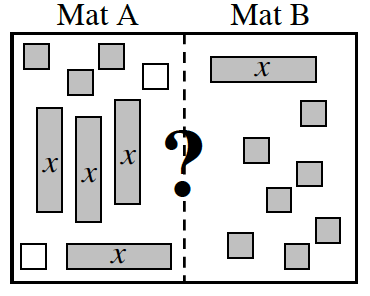
- How is it possible for Mat A to have the greater value?
- How is it possible for Mat B to have the greater value?
- In what other way can Mat A and B be related? Explain.
6-14. Ignacio and Oliver are playing another game with the algebra tiles. After they simplify two new expressions, they are left with the expressions on their mats shown at right. They could not tell which part of the mat is greater just by looking.
- One way to compare the mats is to separate the x‑tiles and the unit tiles on different sides of the mat. Work with your team to find a way to have only x‑tiles on Mat A. Make sure that you are able to justify that your moves are legal.
- Using the same reasoning from part (a), what would you do to have only the variable on Mat B in the Expression Comparison Mat at right?
- Write a short note to Ignacio and Oliver explaining this new strategy. Feel free to give it a name so it is easier for them to remember.
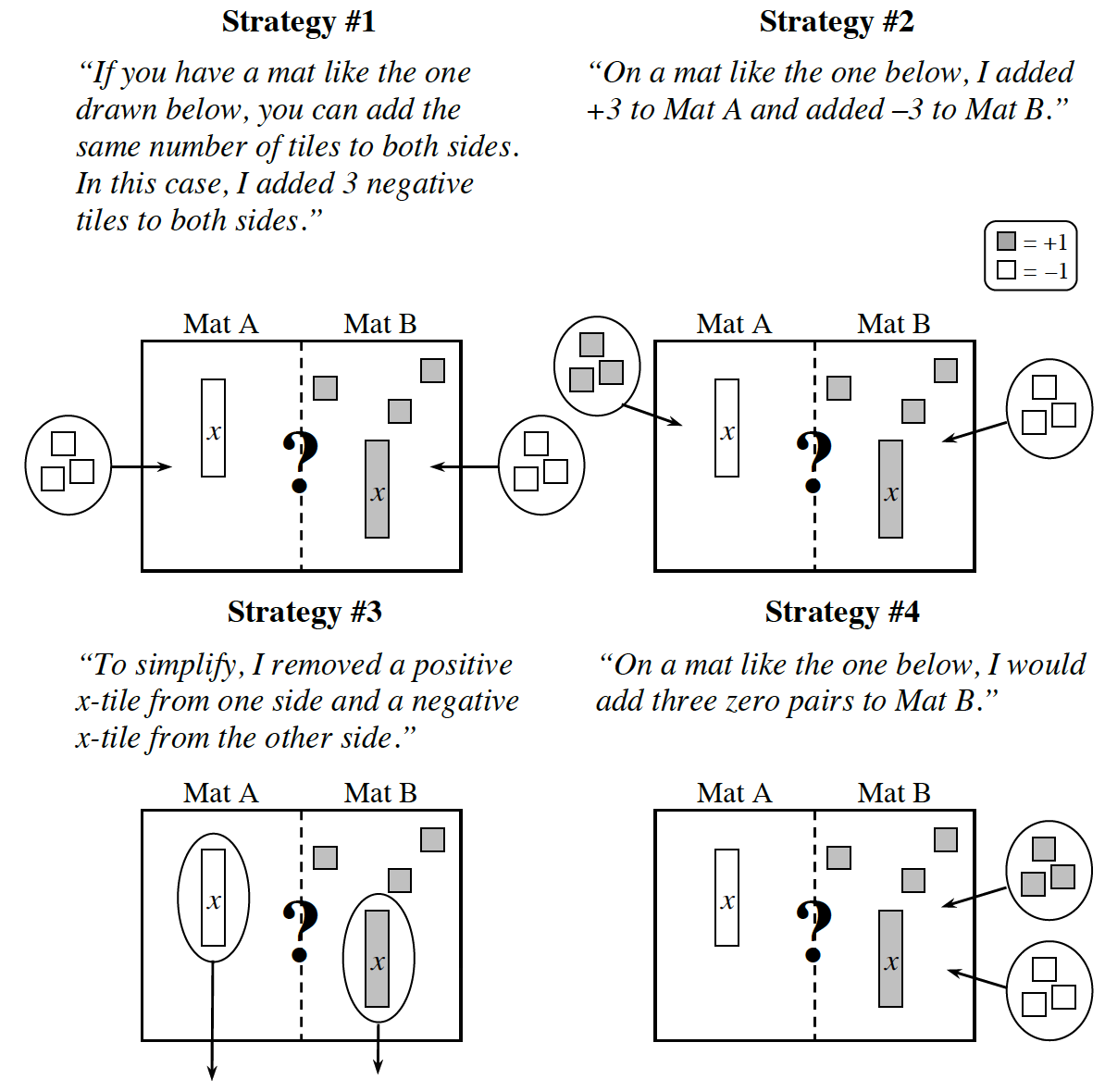
6-15. Ignacio and Oliver are trying to decide if there are other ways to change expressions on the Expression Comparison Mat without affecting which side is greater. They have invented some new strategies and described them below.
Your task: For each of the moves below:
- Build the Expression Comparison Mats on your paper.
- Follow each set of directions for the mat shown in each strategy below.
- Determine if the move in the strategy is valid for maintaining the relationship between the two expressions. Be prepared to justify your response.
6-16. LEARNING LOG
In your Learning Log, summarize the methods that your team and class developed in the last two lessons to simplify expressions on the Expression Comparison Mat. Label your Learning Log entry “Simplifying Expressions (Legal Moves)” and include today’s date.
Assignment: 6.1.2 Homework
[/expand]
[expand title=”Where do the solutions begin an end?”]
You have used Expression Comparison Mats to compare two expressions and have found that sometimes it is possible to determine which expression is greater. In this lesson, you will again compare expressions. This time, you will find the values for the variable that make one expression greater than the other.
6-23. Maria has been recording her work to see which side of an Expression Comparison Mat is greater, but she has been called away. Garth looked at her work, but he cannot figure out what Maria did to get from one step to another.
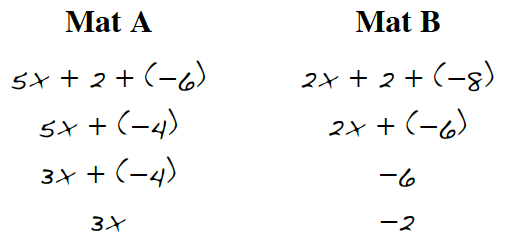Look at Maria’s work above and help Garth by building the expressions on your mat using algebra tiles and simplifying them. Write him a note explaining what Maria did to get from one step to another.
6-24. Compare the expressions 2 + 2x + (−3) and 2x + (−4) + 1 using algebra tiles. Use Maria’s method of recording to show your steps. Make sure you record each step so that your teacher or others could see what you did on your Expression Comparison Mat.
a. Which mat is greater?
b. Use symbols such as <, =, or > to show the relationship between the final expressions on Mat A and Mat B.
6-25. Maria and Garth were playing a game with the algebra tiles. They each grabbed a handful of tiles and put them on the Expression Comparison Mat at right to see whose side had greater value.
Maria said, “I have Mat A and my side has more value.” Garth, who had Mat B, disagreed with her.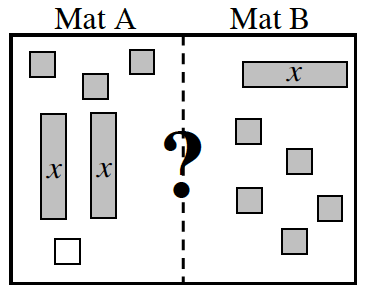
a. Write expressions for Mat A and Mat B. Explore using 6-25 tiles (CPM).
b. Work with your team to simplify the expressions on the Expression Comparison Mat while carefully recording your work for each step on your paper with symbols. Can you tell whose side is greater? Why or why not?
c. With your team, find at least four values for x that would make the expression on Maria’s side (Mat A) greater than the expression on Garth’s side (Mat B). Be prepared to share your values with the class.
d. Any value for x that makes Mat A greater than Mat B is a solution to the inequality 2x + 3 + (–1) > x + 5. This is read, “Two x plus three plus negative one is greater than x plus five.”
6-26. Karla had a hard time keeping track of all of the solutions to the inequality in problem 6-25 in her head. She decided to try to organize her answers. First she needed to know more about the problem.
a. Is there a greatest number that is a solution? Discuss this question with your team and be prepared to share your ideas with the class.
b. Is there a smallest number that is a solution? Again, be prepared to share your team’s thinking with the class.
c. What is special about the point where the solutions end? (This number is called the boundary point.) In other words, what relationship does this number have to the two expressions being compared?
d. Karla was tired of listing so many solutions and wanted a quick way to show all of the solutions to this inequality. She decided to draw a number line like the one below.
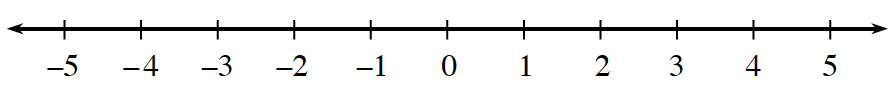
On your own paper, draw a number line such as the one above then follow your teacher’s directions to represent the answer to this question on your number line.
6-27. Now consider the inequality 2x + 5 < 3, which can be read “Two x plus five is less than 3.”
Build the inequality on your Expression Comparison Mat using algebra tiles and record each step on your paper using symbols. Work with your team to describe the least and greatest solutions to the inequality and draw your solution on a number line. Be prepared to justify your ideas.
Assignment: 6.1.3 Homework
[/expand]
[expand title=”How can I find all solutions?”]
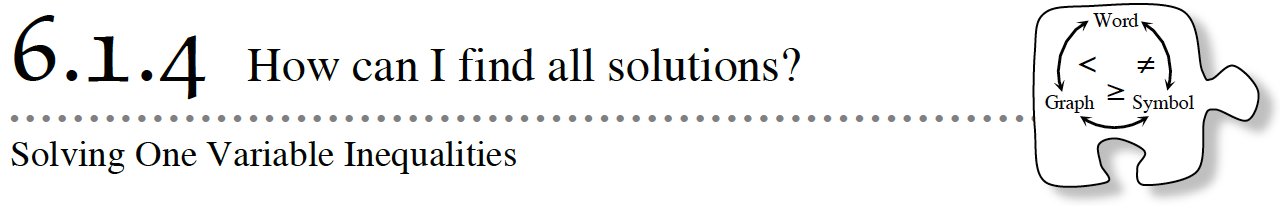
In this lesson, you will work with your team to develop and describe a process for solving linear inequalities. As you work, use the following questions to focus your discussion.
6-35. Jerry and Ken were working on solving the inequality 3x − 1 < 2x. They found the boundary point and Ken made the number line graph shown below.
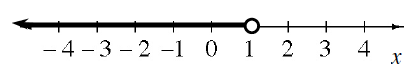
Jerry noticed a problem. “Doesn’t the line at the bottom of the < symbol mean that it includes the equal part? That means that x = 1 is also a solution. How could we show that?”
“Hmmm,” Jerry said. “Well, the solution x = 1 would look like this on a number line. Is there a way that we can combine the two number lines?”
Discuss this idea with your team and be prepared to share your ideas with the class.
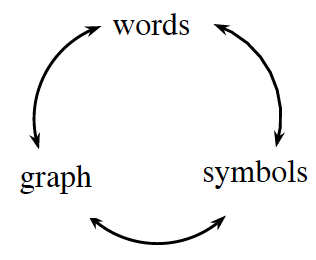6-36. The diagram at right shows three possible ways to represent inequality statements. Review the meanings of the inequality symbols >, <, >, and < with your team. Then, generate the two missing representations from each inequality described in parts (a) through (c) below.
- x < − 1
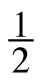
- x is greater than or equal to two.
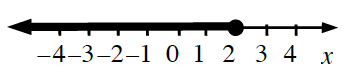
6-37. WHEN IS THE BOUNDARY POINT INCLUDED?
Represent the solution for each of the variables described below as an inequality on a number line and with symbols.
The speed limit on certain freeways is 65 miles per hour. Let x represent any speed that could get a speeding ticket.
You brought $10 to the mall. Let y represent any amount of money you can spend.
To ride your favorite roller coaster, you must be at least five feet tall but less than seven feet tall. Let h represent any height that can ride the roller coaster.
6-38. Ellie was still working on her dollhouse. She has boards that are two different lengths. One long board is 54 inches.
- The length of the short board is unknown. Ellie put three short boards end-to-end and then added her 12-inch ruler end-to-end. The total length was still less than the 54‑inch board. Draw a picture showing how the short and long boards are related.
- Write an inequality that represents the relationship between the short boards and 54 inches shown in your diagram in part (a). Be sure to state what your variable represents.
- What are possible lengths of the short board? Show your answer as an inequality and on a number line.
6-39. Jordyn, Teri, and Morgan are going to have a kite-flying contest. Jordyn and Teri each have one roll of kite string. They also each have 45 yards of extra string. Morgan has three rolls of kite string plus 10 yards of extra string. All of the rolls of string are the same length. The girls want to see who can fly their kite the highest.
- Since Jordyn and Teri have fewer rolls of kite string, they decide to tie their string together so their kite can fly higher. Write at least two expressions to show how much kite string Jordyn and Teri have. Let x represent the number of yards of string on one roll.
- Write an expression to show how much kite string Morgan has. Again, let x be the number of yards of string on one roll.
- How long does a roll of string have to be for Jordyn and Teri to be able to fly their kite higher than Morgan’s kite? Show your answer as an inequality and on a number line.
- How long does a roll of string have to be for Morgan to be able to fly her kite higher than Jordyn and Teri’s kite? Show your answer as an inequality and on a number line.
- What length would the roll of string have to be for the girls’ kites to fly at the same height?
6-40.Additional Challenge: Travis loves trains! Today he is beginning a train ride from Madison, Wisconsin all the way to Seattle, Washington.
Shortly after the train left the station in Madison, Travis fell asleep. When he woke up, it was dark outside and he had no idea how long he had been asleep. A fellow passenger told him they had already passed La Crosse, which is 135 miles from Madison. If the train travels at an average speed of 50 miles per hour, at least how long has Travis been asleep? Represent this problem with an inequality and then solve it.
6-41. LEARNING LOG
Work with your team to describe each step of your process for finding boundary points and deciding what part of the number line to shade. Then write down each step in your Learning Log. Be sure to illustrate your ideas with examples. Title this entry “Finding Boundary Points” and label it with today’s date.
Assignment: 6.1.4 Homework
[/expand]
[expand title=”What values make expressions equal?”]
In the last section, you figured out how to determine what values of x make one expression greater than another. In this lesson you will study what can be learned about x when two expressions are equal. As you work today, focus on these questions:
6-48. CHOOSING A PRICE PLAN
Sandeep works at a bowling alley that currently charges players $3 to rent shoes and $4 per game. However, his boss is thinking about charging $11 to rent shoes and $2 per game.
a. If a customer rents shoes and plays two games, will he or she pay more with the current price plan or the new price plan? Show how you know.
b. If the customer bowls 7 games, which price plan is cheaper?
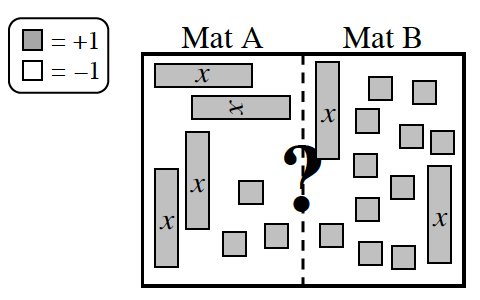
6-49. WILL THEY EVER BE EQUAL?
Sandeep decided to represent the two price plans from problem 6-48 with the expressions below, where x represents the number of games bowled. Then he placed them on the Expression Comparison Mat shown at right.
6-50. SOLVING FOR X
When the expressions on each side of the comparison mat are equal, they can be represented on a mat called an Equation Mat. Obtain a Lesson 6.2.1 Resource Page and algebra tiles from your teacher. Now the “=” symbol on the central line indicates that the expressions on each side of the mat are equal.
a. Build the equation represented by the Equation Mat at right on your own mat using algebra tiles.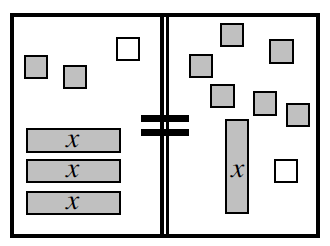
b. On your paper, record the original equation represented on your Equation Mat.
c. Simplify the tiles on the mat as much as possible. Record what is on the mat after each legal move as you simplify each expression. What value
of x will make the expressions equal?
6-51. Amelia wants to solve the equation shown on the Equation Mat at right. After she simplified each expression as much as possible, she was confused by the tiles that were left on the mat.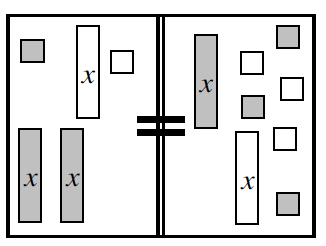
a. What was Amelia’s original equation?
b. Remove any zero pairs that you find on each side of the Equation Mat. What happens?
c. What is the solution to this equation? That is, what value of x makes this equation true? Explain your reasoning.
6-52. Amelia now wants to solve the equation 2x + 2 + (−3) = 5x + 8. Help her find the value of x that makes these expressions equal. Be sure to:
a. Build the expressions using algebra tiles on your Equation Mat.
b. Draw the mat on your paper.
c. Simplify the mat to help you figure out what value of x makes this equation true. Be sure to record your work in symbols on your paper.
6-53.Additional Challenge: Try the number puzzle below.
Pick any number, add 20, multiply your answer by 2, and subtract 30 from the result. Then, divide your answer by 2 and subtract your original number.
a. What is the result?
b. Try it with several different numbers. Is the result the same every time?
c. Using a variable (such as n) to represent the starting number, show why the final result is always the number 5.
Assignment: 6.2.1 Homework
[/expand]
[expand title=”How do I know that it is correct?”]
Sometimes a lot can depend on the solution of a problem. For example, when businesses calculate the cost of packaging and shipping a product, they need to come up with an accurate value. If they miscalculate by only $0.01 per package but ship one million packages per year, this small miscalculation could be costly.
Solving a problem is one challenge. However, once it is solved, it is important to have ways to know whether the solution you found is correct. In this lesson, you will be solving equations and finding ways to determine whether your solution makes the equation true.
6-60. Chen’s sister made this riddle for him to solve: “I am thinking of a number. If you add two to the number then triple it, you get nine.”
a. Build the equation on an Equation Mat using algebra tiles. What are two ways that Chen could write this equation?
b. Solve the equation and show your work by writing the equation on your paper after each legal move.
c. When Chen told his sister the mystery number in the riddle, she said he was wrong. Chen was sure that he had figured out the correct number. Find a way to justify that you have the correct solution in part (b).
6-61. Now solve the equation 4(x + 3) = 8. Remember to:
- Build the equation on your Equation Mat with algebra tiles.
- Simplify the equation using your legal moves.
- Record your work on your paper.
- Solve for x. That is, find the value of x that makes the equation true.
6-62. CHECKING YOUR SOLUTION
When you solve an equation that has one solution, you get a value for the variable. But how do you know that you have done the steps correctly and that your answer “works”?
a. Look at your answer for problem 6-61. How could you verify that your solution is correct and convince someone else? Discuss your ideas with your team.
b. When Kelly and Madison compared their solutions for the equation 2x − 7 = −2x + 1, Kelly got a solution of x = 2 and Madison got a solution of x = −1. To decide if the solutions were correct, the girls decided to check their answers to see if they made the expressions equal.
Finish their work below to determine if either girl has the correct solution.
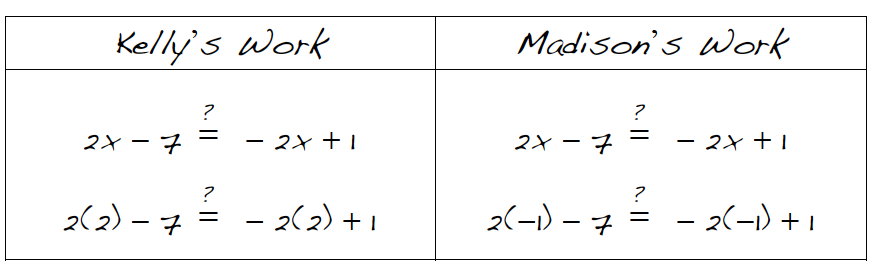
c. When checking, Kelly ended up with −3 = −3. Does this mean that her answer is correct or incorrect? And if it is correct, does this mean the solution is x = −3 or x = 2? Explain.
d. Go back to problem 6-61 and show how to check your solution for that problem.
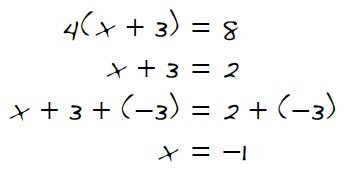
6-63. Kelly solved the equation 4(x + 3) = 8 from problem 6-61. Her work is shown at right.
a. If 4(x + 3) = 8, does x + 3 have to equal 2? Why?
b. What did Kelly do to remove the 3 unit tiles from the left side of the equation? Does this move affect the equality?
c. If Kelly were solving the equation 3(x − 5) = 9, what might her first step be? What would she have after that step? You may want to build this equation on an Equation Mat to help make sense of her strategy.
6-64. Now practice this new solving skill by building each of the equations below with algebra tiles, solving for x, and checking your solution for each equation. Record your work. (PDF Worksheet: 6.2.2 Problems)
- 4(x + 1) + 1 + (−x) = 10 + x
- −1 + 2x − x = x − 8 + (−x)
- 5 + 2(x − 4) = 4x + 7
- 9 − 3x = 1 + x
- 3x + 3 − x + 2 = x + 5
- 4 = 3(2x + 1) − 11
Assignment: 6.2.2 Homework
[/expand]
[expand title=”How can I record it?”]
In this lesson, you will continue to improve your skills of simplifying and solving more complex equations. You will develop ways to record your solving strategies so that another student can understand your steps without seeing your Equation Mat. Consider these questions as you work today:
6-71. Gene and Aidan were using algebra tiles to solve equations. Aidan was called away.
Help Gene finish by completing the table shown below and on the Lesson 6.2.3 Resource Page.
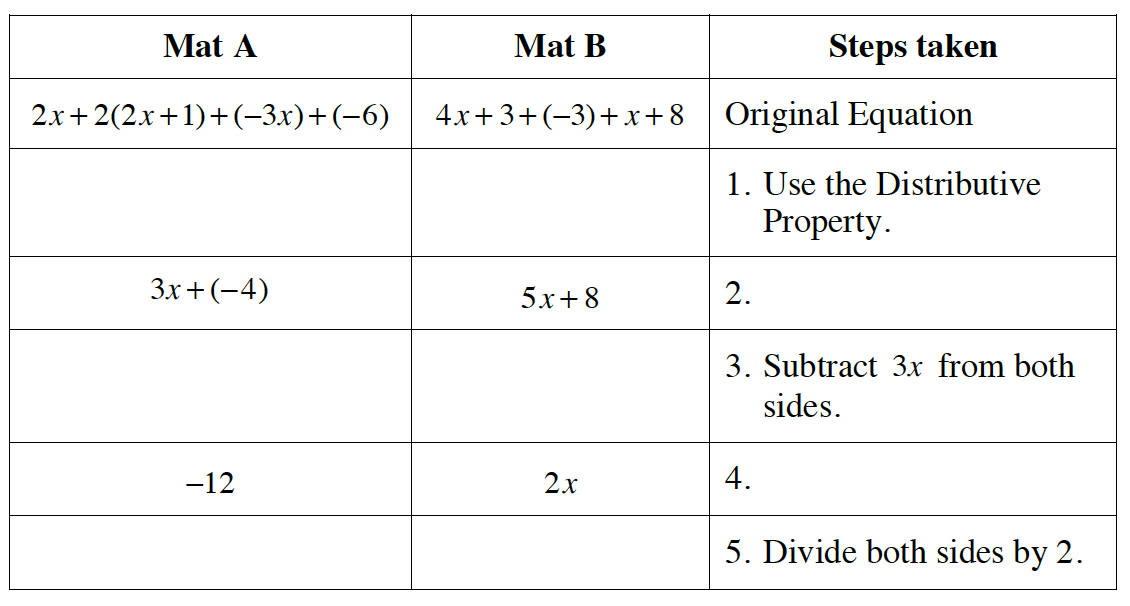
6-72. Aidan was frustrated that he needed to write so much when solving an equation. He decided to come up with a shortcut for recording his work to solve a new equation.
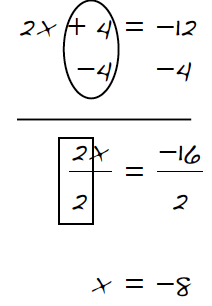As you look at Aidan’s recording at right of how he solved 2x + 4 = −12 below, visualize an Equation Mat with algebra tiles. Then answer the questions for each step below.
a. What legal move does writing –4 twice represent?
b. What legal move does circling the +4 and the –4 represent?
c. What does the box around the 
represent?
d. Why did Aidan divide both sides by 2?
e. Check Aidan’s solution in the original equation. Is his solution correct?
6-73. The method of recording the steps in the solution of an equation is useful only if you understand what operations are being used and how they relate to the legal moves on your Equation Mat.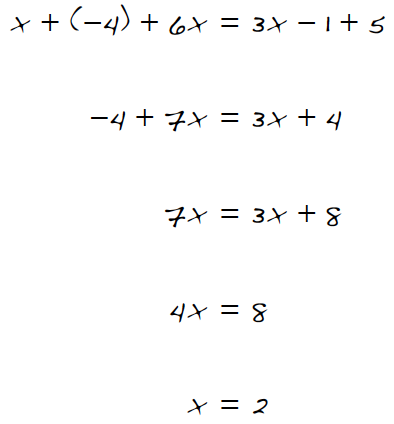 
Find the work shown at right on your resource page for this lesson.
a. For each step in the solution, add the missing work below each line that shows what legal moves were used. You may want to build the equation on an Equation Mat.
b. Check that the solution is correct.
6-74. For each equation below, solve for x. You may want to build the equation on your Equation Mat. Record your work in symbols using Aidan’s method from problem 6‑72. Remember to check your solution.
a. −2x + 5 + 2x − 5 = −1 + (−1) + 6x + 2
b. 3(4 + x) = x + 6
6-75. Oliver is building a train depot for his model railroad. As his final step, he needs to apply rain gutters around the roof of the rectangular building. He has 52 cm of rain gutters. The length of the depot is 19 cm. Explore how Oliver can find the width of the depot by answering the questions below.
a. Find the width of the depot using arithmetic (that is, solve the problem without using any variables). Record your steps.
b. Use w to represent the width of the depot. Write an algebraic equation that shows the perimeter is 52 cm, and solve your equation. Record your steps.
c. Which method, the arithmetic or algebraic, did you prefer? Why?
6-76. For each equation below, solve for x. You may want to build the equation on your Equation Mat. Record your work and check your solution.
a. 5x + (−x) − 1 = 11 − 2x
b. 3(−x + 2) + x − 1 = −x − 3
6-77. Genny is confused. She simplified an equation and ended up with the mat shown below. What is the value of x?
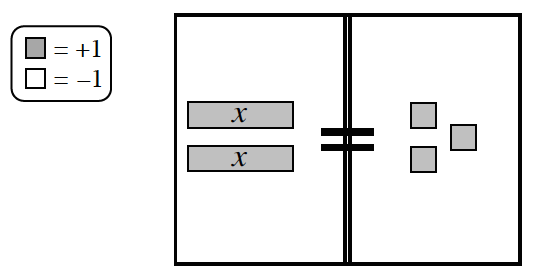
6-78. Maggie’s mom agrees to let Maggie buy small gifts for some of her friends. Each gift costs $4. Maggie’s mom gave her a budget of $19. When Maggie went online to order the gifts, she discovered there was a $7 shipping fee no matter how many gifts she bought.
- Use arithmetic (without variables) to determine how many gifts Maggie can buy. Record your steps.
- Write an algebraic equation to determine how many gifts Maggie can buy with $19, and solve your equation. Record your steps.
- Compare and contrast the two methods of solving the problem.
6-79. Your teacher will explain the way you will be working on solving the equations below for x. You may want to build the equations on your Equation Mat. Record your work and check your solution.
- 2(x + 1) + 3 = 3(x − 1)
- −2x − 2 = 3(−x + 2)
- 3 + 4(2 − x) = 3x + (−x) − 7
- 6(3 − x) + (−20) = 10 + 3(4x + 2)
6-80. LEARNING LOG
In your Learning Log, explain what it means to solve an equation. What is a solution? Be sure to give an example. Title this entry “Solving Equations and Checking Solutions” and include today’s date.
Assignment: 6.2.3 Extra Practice Problems
[/expand]
[expand title=”How can I model it?”]
Engineers investigate practical problems to improve people’s quality of life. To investigate solutions to problems, they often build models. These models can take various forms. For example, a structural engineer designing a bridge might build a small replica of the bridge. Civil engineers studying the traffic patterns in a city might create equations that model traffic flows into and out of a city at different times.
In this lesson, you will be building equations to model and solve problems based on known information. As you work today, keep the following questions in mind.
Connections between the words in the problem and the relationships in your table and/or equation. Connections can be made with arrows, colors, symbols, and/or labels.
- Variables that are defined completely.
- An equation to represent the problem.
- Your solution to the problem.
- The answer declared in a sentence.
- An estimation verifying that the total of your solutions is reasonable.
Begin by solving one of the problems below and writing an equation. Make sure to define the variable you use and answer the question(s) being asked. Using the 5-D Process, including numerical trials, may be helpful.
- Hong Kong’s tallest building, Two International Finance Center, is 88 stories tall. The former Sears Tower in Chicago is eight stories taller than the Empire State Building in New York City. If all of the buildings were stacked on top of each other, the combined heights would have 300 stories. How many stories does the Sears Tower have?
- Have you ever driven or walked across a suspension bridge? There are many suspension bridges in the world of different lengths that allow people to travel across rivers, bays, and lakes.The Mackinac Bridge in Michigan is 1158 meters long. The Tsing Ma Bridge in Hong Kong is 97 meters longer than the Golden Gate Bridge in California. Together, all three bridges have a length of 3815 meters. How long is the Tsing Ma Bridge?
Elevations found in the United States range from California’s Death Valley at 282 feet below sea level to Alaska’s Mount McKinley, also known as Denali, at 20,320 feet above sea level.The highest elevation in Delaware is 106 feet higher than the highest elevation in Florida. Louisiana’s highest elevation is 190 feet higher than Florida’s highest elevation. If you climbed from sea level to the highest points in Delaware, Florida, and Louisiana, you would only climb 1331 feet. How high is the highest elevation in each of the three states?- Most states in the United States are divided into counties. Some counties are very large, while some are very small. Different states have different numbers of counties. Pennsylvania has five less than twice as many counties as Oregon. Florida has one less county than Pennsylvania. Together, the three states have 169 counties. How many counties does each of these three states have?
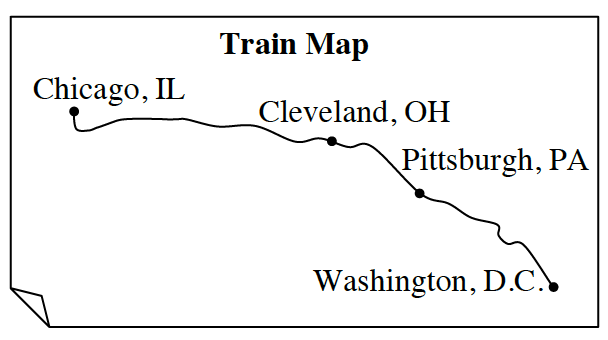A train from Washington, D.C. to Chicago stops first in Pittsburgh and then in Cleveland. The distance from Washington, D.C. to Pittsburgh is 30 miles less than twice the distance from Pittsburgh to Cleveland. The distance from Cleveland to Chicago is 220 miles more than the distance between Pittsburgh and Cleveland. If the entire train ride is 710 miles, how far is the train ride from Cleveland to Chicago?
[/expand]
[expand title=”Is there always a solution?”]
Are all equations solvable? Are all solutions a single number? Think about this: Annika was born first, and her brother William was born 4 years later. How old will William be when Annika is twice his age? How old will William be when Annika is exactly the same as his age?
In this lesson, you will continue to practice your strategies of combining like terms, removing zeros, and balancing to simplify and compare two expressions. You will also encounter unusual situations where the solution may be unexpected. As you work today, focus with your team on these questions:
6-116. Many students believe that every equation has only one solution. However, in the introduction to this lesson you might have noticed that if Annika was four years older than her brother, William, they could never be the same age. Some situations have one solution, others have no solution, and still others have all numbers as solutions.
For each of the following equations, reason with your team to decide if there is “One solution,” “No solution,” or “All numbers are solutions.” If there is a single number solution, write it down. If you are not sure how many solutions there are, have each member of your team try a number to see if you can find a value that makes the equation work.
- x = x
- x + 1 = x
- x = 2x
- x + x = 2 + x
- x + x = x − x
- x + x = 2x
- x · x = x2
- x − 1 = x
6-118. SPECIAL CASES, Part One
Use the equation 8 + x + (−5) = (−4) + x + 7 to complete parts (a) through (c).
a. Build the equation on your Equation Mat using algebra tiles and simplify it as much as possible. Record your steps and what you see when you have simplified the equation fully. Draw a picture of your final mat.
b. Have each member of your team test a different value for x in the original equation, such as x = 0, x = 1, x = −5, x = 10, etc. What happens in each case?
c. Are there any solutions to this equation? If so, how many?
6-119. SPECIAL CASES, Part Two
Use the equation x + x + 2 = 2x to complete parts (a) through (c).
a. Build the equation on your Equation Mat using algebra tiles and simplify it as much as possible. Record your steps and what you see when you have simplified the equation fully. Draw a picture of your final mat.
b. Have each member of your team test a different value for x in the equation, such as x = 0, x = 1, x = −5, x = 10, etc. What happens? Is there a pattern to the results you get from the equation?
c. Did you find any values for x that satisfied the equation in part (a)? When there is an imbalance of units left on the mat (such as 2 = 0), what does this mean? Is x = 0 a solution to the equation?
6-120. Keeping these special cases in mind, continue to develop your equation-solving strategies by visualizing and solving each equation below. Remember to build each equation on your mat, if needed, to simplify as much as possible, and to solve for x. Identify if one number is the solution, if any number is the solution, or if there is no solution. Record your steps.
- −x + 2 = 4
- −3 + x = 2(x + 3)
- 5x + 3 + (−x) = 2x + 1 + 2x + 3
- 3x + 7 + (−x) + −2 = 2x + 5
- 4 + −3x = 2
- 3x + 3 = 4 + x + (−1)
6-121. Additional Challenge: For each of the parts below, create your own equation (involving at least three steps) that has the given solution.
- x = –2
- x =
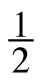
- no solution
- all numbers
Assignment: 6.2.6 Homework
[/expand]
[expand title=”Assessment”]
[/expand]