Essential Question: How is finding an associated rate or unit rate helpful when making comparisons between quantities?
7.RP.2
[expand title=”Lesson”]
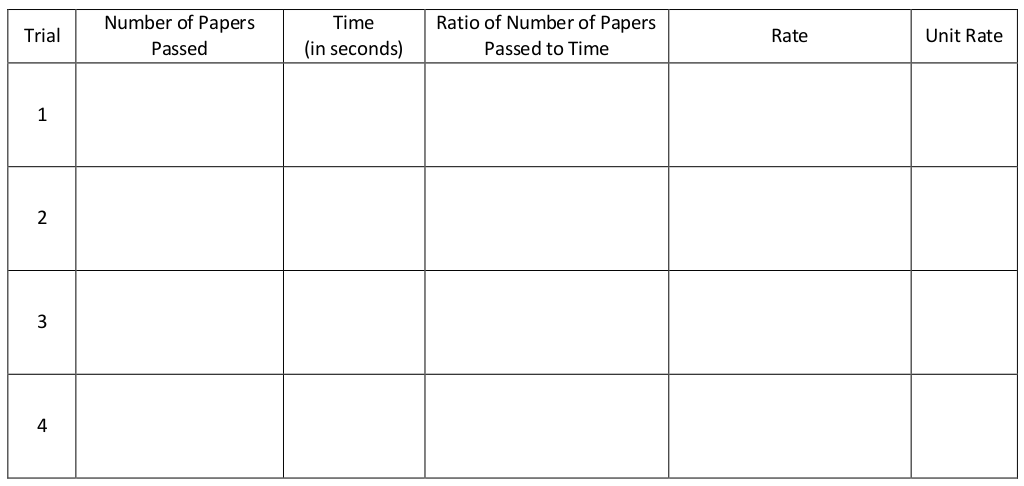
S.1 Example 1: How fast is our class?
Discussion
- How will we measure our rate of passing out papers?
- What quantities will we use to describe our rate?
S.2 Example 2: Which is a better buy?
Value-Mart is advertising a Back-To-School sale on pencils. A pack of 30 sells for $7.97 whereas a 12-pack of the same brand cost $4.77. Which is a better buy? How do you know?
Discussion
- How did you determine if the ratios are equivalent?
- Which is a better buy? Why?
[/expand]
[expand title=”Summary”]
How is finding an associated rate or unit rate helpful when making comparisons between quantities?
- The unit rate tells the quantity of one unit required for just one of another unit. For example, a unit price of 0.4 means 1 juice box from a 6-pack costs $0.4.
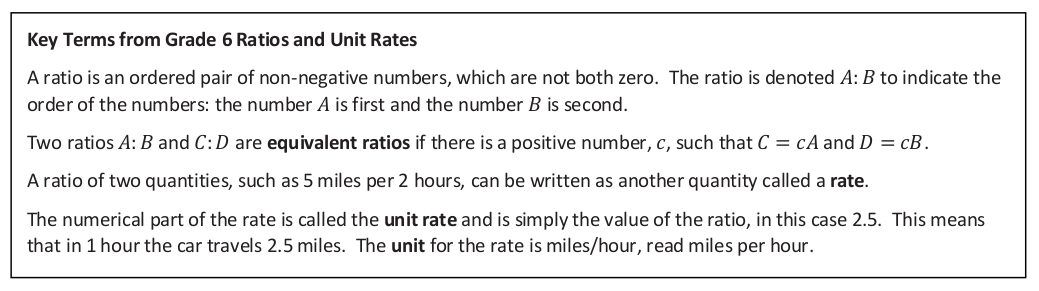
Unit Rate is a useful way of comparing ratios and rates containing different units. Unit rate allows us to examine units of one quantity per 1 unit of the second quantity. Unit rates allow us to convert from one unit to another, such as 12 inches per foot, or
A very common example of a unit rate is Miles Per Hour (MPH). As a ratio, this is the number of miles traveled in 1 hour. Notice how one of the units is 1, making this a unit rate.
[/expand]
[expand title=”Problems”]
Problem Set
1. Find each rate and unit rate.
a. 420 miles in 7 hours
b. 360 customers In 30 days
c. 40 meters in 16 seconds
d. $7.96 for 5 pounds
2. Write three ratios that are equivalent to the one given: 18 right-handed students for every 4 left-handed students.
3. Mr. Rowley has 16 homework papers and 14 exit tickets to return. Ms. Rivera has 64 homework papers and 60 exit
tickets to return. For each teacher, write a ratio to represent the number of homework papers to number of exit
tickets they have to return. Are the ratios equivalent? Explain.
4. Jonathan’s parents told him that for every 5 hours of homework or reading he completes, he will be able to play 3
hours of video games. His friend Lucas’s parents told their son that he can play 30 minutes for every hour of
homework or reading time he completes. If both boys spend the same amount of time on homework and reading
this week, which boy gets more time playing video games and how do you know?
5. At Euclid Middle School, of the 30 girls who tried out for the lacrosse team, 12 were selected and of the 40 boys who
tried out, 16 were selected. Are the ratios of number of students on the team to number of student trying out the
same for both boys and girls? How do you know?
6. Devon is trying to find the unit price on a 6-pack of energy drinks on sale for $2.99. His sister says that at that price,
each energy drink would cost just over $2.00. Is she correct and how do you know? If she is not, how would
Devon’s sister find the correct price?
7. Each year Lizzie’s school purchases student agenda books, which are sold in the school store. This year, the school
purchased 350 books at a cost of $1,137.50. If the school would like to make a profit of $1,500 to help pay for field
trips and school activities, what is the least amount they can charge for each agenda book? Explain how you found
your answer.
[/expand]
[expand title=”Exit Ticket”]
Tillman the Skateboarding English Bulldog
Watch the video clip of Tillman the English Bulldog, the Guinness World Record holder for Fastest Dog on a Skateboard.
1.At the conclusion of the video, your classmate takes out his or her calculator and says, “Wow that was amazing!
That means the dog went about 5 meters in 1 second!” Is your classmate correct, and how do you know?
2.After seeing this video, another dog owner trained his dog, Lightning, to try to break Tillman’s skateboarding record.
Lightning’s fastest recorded time was on a 75meter stretch where it took him 15.5 seconds. Based on this data, did
Lightning break Tillman’s record for fastest dog on a skateboard? Explain how you know.
[/expand]